Magnetic properties of materials

First part:

Definition of fundamental quantities

Classification of materials

Calculation of atomic susceptibilities

Langevin diamagnetism 

Paramagnetism of insulators 

Hund Rules 

Rare-earth ions 

Iron-group ions 

Paramagnetism of conduction electrons

Diamagnetism 

To download the file click on the link below:

http://unlcms.unl.edu/cas/physics/tsymbal/teaching/SSP-927/Section%2015_Magnetic_Properties_1.pdf

Second part:

Ferromagnetism

Spin waves 

Thermal excitation of magnons 

Ferrimagnetism 

 Antiferromagnetism

Magnetic domains in ferromagnetic materials

    Magnetization curve

    Magnetic domains

           1. Exchange energy

           2. Magnetostatic energy

           3. Anisotropy energy

           4. Magnetoelastic energy

   Domain walls 

   The effect of applied field and domain wall motion 

To download the file click on the link below:

http://unlcms.unl.edu/cas/physics/tsymbal/teaching/SSP-927/Section%2016_Magnetic_Properties_2.pdf

Ferrimagnetism 

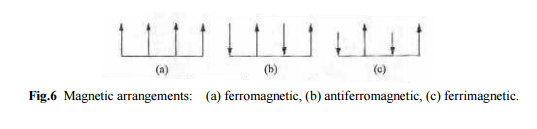
The Heisenberg model (11) leads to ferromagnetism, if the constant J is positive. The parallel-aligned state will then have a lower energy then the antiparallel state. The negative constant in Eq.(11) leads to antiferromagnetism or ferrimagnetism. Figure 6b illustrates an antiferromagnetic arrangement, in which the dipoles have equal moments, but adjacent dipoles point in opposite directions. Thus the moments balance each other, resulting in a zero net magnetization. Another type of arrangement commonly encountered is the ferrimagnetic pattern shown in Fig.6c. Neighboring dipoles point in opposite directions, but since in this case the moments are unequal, they do not balance each other completely, and there is a finite net magnetization. Now we discuss the ferrimagnetic arrangement.

The most familiar example of a ferrimagnetic material is magnetite, Fe3O4. More explicitly, the chemical composition is FeO⋅Fe2O3, showing that there are two types of iron ions: ferrous (doubly charged), and ferric (triply charged). The compound crystallizes in the spinel structure. The unit cell contains 56 ions, 24 of which are iron ions and the remainder oxygen. The magnetic moments are located on the iron ions. If we study the unit cell closely, we find that the Fe ions are located in either of two different coordinate environments: A tetrahedral one, in which the Fe ion is surrounded by 4 oxygen ions, and an octahedral one, in which it is surrounded by 6 oxygen ions. Of the 16 ferric ions in the unit cell, 8 are in one type of position and 8 are in the other. Furthermore, the tetrahedral structure has moments oriented opposite to those of the octahedral one, resulting in a complete cancellation of the contribution of the ferric ions. The net moment therefore arises entirely from the 8 ferrous ions which occupy octahedral sites. Each of these ions has six 3d electrons, whose spin orientations are ↑↑↑↑↑↓. Hence each ion carries a moment equal to 4 Bohr magneton.

There are many other materials which have ferrimagnetic properties. An important class of magnetic oxides is known as ferrites. The usual chemical formula of a ferrite is MO⋅Fe2O3, where M is a divalent cation, often Zn, Cd, Fe, Ni, Cu, Co, or Mg.